This website is built with WordPress. This relies on cookies for basic management tasks and site functionality. To learn more about our policies, visit our Privacy Policy

Man Ray, Mathematical Object, 1934-35. ©Man Ray Trust / Artist Rights Society (ARS), New York, ADAGP Paris
Categories
Reviews

Man Ray: Human Equations: A Journey from Mathematics to Shakespeare

BY Sarah Boxer, February 27, 2015

caption
Man Ray, Mathematical Object, 1934-35. ©Man Ray Trust / Artist Rights Society (ARS), New York, ADAGP Paris

In Man Ray’s own introduction to the series of paintings he named “The Shakespearean Equations,” he had the good and the bad sense to quote a friendly critic of his art: Andre Breton had warned Man Ray that by showing his pieces next to the mathematical objects that had inspired them, the art would risk seeing “its realization definitely outclassed.” Breton was right. You can’t beat mathematics for stark beauty. 

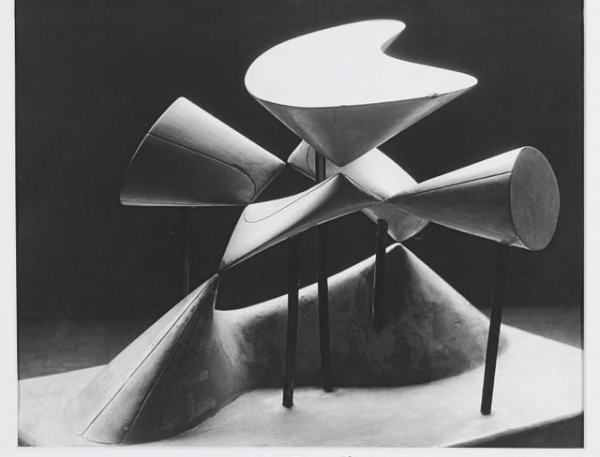
The most fascinating, uncanny objects in the exhibition Man Ray – Human Equations: A Journey From Mathematics to Shakespeare, at the Phillips Collection through May 10, are not the ones made by the artist but rather the mathematical objects that they’re based on, models made out of white plaster, papier-mache, wood, thread, string, and metal, from the Institut Henri Poincaré in Paris. These models, made around 1900, are simply beautiful and, if you’re not a mathematician, simply incomprehensible. 

Here’s the back-story: In the 1930s, Max Ernst encouraged Man Ray, a fellow Surrealist, to visit these models of mathematical equations at the Institut Poincaré. One of the models, a 3-D illustration of a “Kummer Surface with Eight Real Double Points,” for instance, is an arrangement of Jean-Arp-like shapes – conical objects with some flat facets and some curvaceous bites taken out of them. At first, Man Ray merely photographed the models, using dramatic lighting to bring out their angles, shadows, and grooves. 

caption
Man Ray, Shakespeare Equation, Twelfth Night, 1948. ©Man Ray Trust / Artist Rights Society (ARS), New York, ADAGP Paris

But Man Ray went one step, sometimes two steps, too far. In the late 1940s, long after he’d left occupied France and moved to the United States, he revisited the photographs he’d taken in the 1930s and made paintings based on them – the “Human Equations.” And once he had finished the paintings, he gave some of them Shakespearean titles; these were his “Shakespearean Equations.” For instance, his painting based on the “Kummer Surface” model seems to show a tawny, flat-headed figure running with his arms thrown out; this becomes “King Lear.” For another painting based on a mathematical model, which resembles a man’s starched shirt front with holes gouged out, he adds in the figure of an upside-down chair leg with a guilty-looking caster as a head; this becomes “Julius Caesar.” As Breton all but predicted, the comparison of gorgeous, uncanny mathematical models with Surrealist painting does Surrealist painting no favor at all.